Natural Frequency of Free Torsional Vibrations

Consider a uniform shaft of negligible mass rigidly fixed at its upper end and carrying a disc at its lower end, as shown in Fig. If the disc is given a twist about its vertical axis and then released, it will start twisting and untwisting about the axis, which is known as torsional vibrations.

Let,

m = Mass of the disc in kg,
k = Radius of gyration of the disc in metres,
I = mk² = Mass moment of inertia of the disc in kg-m²
θ = Angular displacement of the disc from its equilibrium at any instant in radians, and
q = Torsional stiffness of the shaft or torque required to twist the shaft through one radian in N-m.

Equilibrium (or Newton’s) Method

The various torques acting on the disc, at any instant, are:

Accelerating torque $=\mathrm{I} \frac{d^{2} \theta}{d t^{2}} \quad$ (say, clockwise, when seen from top)

∴ Inertia torque $=\mathrm{I} \frac{d^{2} \theta}{d t^{2}} \quad$ (Anticlockwise, when seen from top) and Restoring (or spring) torque = q.θ (Anticlockwise, when seen from top)

Therefore, according to D’ Alembert’s principle,

Inertia torque + Σ External torques = 0

\qquad \begin{aligned} \text { I } \frac{d^{2} \theta}{d t^{2}}+q \theta &=0 \\ \frac{d^{2} \theta}{d t^{2}}+\frac{q}{\mathrm{I}} \theta &=0 \end{aligned}

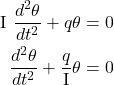
This is the fundamental or differential or governing equation of undamped free torsional vibrations.

We know that the fundamental equation of SHM,

\frac{d^{2} \theta}{d t^{2}}+\omega^{2} \theta=0

Comparing equations before and (i), we get

\omega^{2}=\frac{q}{I}

\omega=\sqrt{\frac{q}{I}}

∴ Time period, $t_{\mathrm{P}}=\frac{2 \pi}{\omega}=2 \pi \sqrt{\frac{\mathrm{I}}{q}}$

and Natural frequency, $f_{n}=\frac{1}{t_{p}}=\frac{1}{2 \pi} \sqrt{\frac{q}{\mathrm{I}}}$

Note :

The natural frequency of torsional vibration can also be determined by the energy and Rayleigh methods (in the similar manner as discussed in longitudinal vibrations,
Torsional stiffness (q) : Torsional stiffness is nothing but the torque required to produce unit angular deflection.
Mathematic, $\quad q=\frac{\mathrm{T}}{\theta}=\frac{\mathrm{C} \mathrm{J}}{l}\left[\because \text { From torsion equation } \frac{\mathrm{T}}{\mathrm{J}}=\frac{\mathrm{C} \theta}{l}\right] \\$ where q = Torsional stiffness N-m,
T = Applied torque in N-m
θ = Angular displacement of the disc in radians,
C = Modulus of rigidity for the shaft material in N/m,
J = Polar moment of inertia in $\mathrm{m}^{4}=\frac{\pi}{32} d^{4}$
d = Diameter of the shaft in metres, and
l = Length of the shaft in metres.
Substituting $q=\frac{\mathrm{CJ}}{l}$ in equation before, we get $f_{n}=\frac{1}{2 \pi} \sqrt{\frac{q}{\mathrm{I}}}=\frac{1}{2 \pi} \sqrt{\frac{\mathrm{CJ}}{\mathrm{I} l}}$
Analogy between linear and torsional vibrations: In fact, the concept, analysis, and expressions of torsional vibrations are very much similar to linear (longitudinal and transverse) vibrations. Hence, for easy and better understanding, the analogy between linear and torsional vibrations are present in Table.

Parameters	Linear Vibration		Torsional Vibration
Parameters	Symbol	Unit	Symbol	Unit
Displacement	𝑥	m	θ	rad
Velocity	v or $\dot{x}$	m/sec	ω or $\dot{\theta}$	rad/sec
Acceleration	a or $\ddot{x}$	m/sec	α or $\ddot{\theta}$	rad/sec²
Mass	m	kg	I	kg-m²
Inertia force or torque	ma	N	$\mathrm{I} \ddot{\theta}=\mathrm{I} \alpha$	N-m
Stiffness	s	N/m	q	Nm/rad
Natural frequency	$f=\frac{1}{2 \pi} \sqrt{\frac{s}{m}}$	Hz	$f=\frac{1}{2 \pi} \sqrt{\frac{q}{\mathrm{I}}}$	Hz