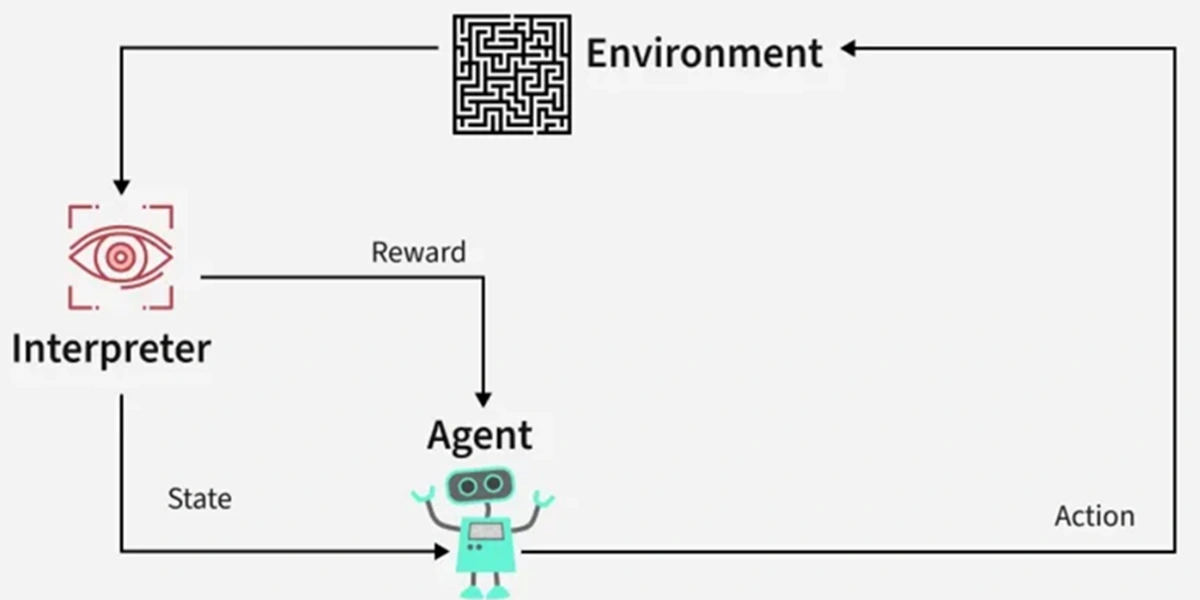
In a world characterized by intricate relationships and complex systems, the importance of nonlinear analysis cannot be overstated. While linear models have long been the go-to approach for understanding various phenomena, they often fall short when confronted with the true complexity of real-world applications. This is where nonlinear analysis steps in, offering powerful tools and techniques to unravel the mysteries of systems that don’t follow simple, proportional relationships.

Understanding Nonlinear Analysis
Before delving into its applications, let’s first grasp what nonlinear analysis entails. At its core, nonlinear analysis is a branch of mathematics and engineering that deals with systems whose output is not directly proportional to their input. In other words, doubling the input doesn’t necessarily double the output, and the system’s behavior can be highly sensitive to initial conditions.
Nonlinear systems are characterized by phenomena such as:
1. Chaotic behavior
2. Multiple equilibria
3. Limit cycles
4. Bifurcations
5. Emergent properties
These characteristics make nonlinear systems both fascinating and challenging to study. Traditional linear methods often fail to capture the rich dynamics of such systems, necessitating more advanced analytical techniques.
Real-World Applications of Nonlinear Analysis
The power of nonlinear analysis lies in its ability to model and predict complex behaviors across a wide range of fields. Let’s explore some of the most impactful applications:
1. Climate Science and Weather Forecasting
Perhaps one of the most well-known applications of nonlinear analysis is in the field of climate science and weather forecasting. The Earth’s climate system is inherently nonlinear, with countless variables interacting in complex ways. Nonlinear models help scientists:
– Predict extreme weather events
– Understand climate change patterns
– Model ocean currents and atmospheric dynamics
The famous “butterfly effect,” coined by Edward Lorenz, emerged from his work on nonlinear weather models. It illustrates how small changes in initial conditions can lead to drastically different outcomes in chaotic systems.
2. Financial Markets and Economic Systems
Financial markets are another prime example of complex, nonlinear systems. Stock prices, exchange rates, and economic indicators often exhibit chaotic behavior that defies simple linear analysis. Nonlinear techniques are employed to:
– Analyze market trends and volatility
– Develop trading strategies
– Assess and manage financial risks
– Model economic growth and business cycles
Techniques like fractal analysis and neural networks, rooted in nonlinear mathematics, have become invaluable tools for financial analysts and economists.
3. Biological Systems and Population Dynamics
Life itself is a testament to the power of nonlinear systems. From the molecular level to entire ecosystems, biological processes are governed by complex, nonlinear interactions. Applications include:
– Modeling predator-prey relationships
– Understanding the spread of infectious diseases
– Analyzing neural networks in the brain
– Studying population growth and extinction patterns
The famous Lotka-Volterra equations, which model predator-prey dynamics, are a classic example of nonlinear differential equations in biology.
4. Engineering and Control Systems
In the world of engineering, nonlinear analysis plays a crucial role in designing and optimizing various systems:
- Structural engineering: Analyzing the behavior of materials under extreme conditions
- Aerospace engineering: Modeling flight dynamics and spacecraft trajectories
- Control systems: Designing robust controllers for nonlinear processes
- Robotics: Developing adaptive control algorithms for complex movements
Nonlinear control theory has revolutionized the field of robotics, enabling the creation of more agile and responsive machines.
5. Signal Processing and Communications
As our world becomes increasingly connected, the importance of efficient and reliable communication systems grows. Nonlinear analysis contributes to:
– Optimizing data compression algorithms
– Enhancing signal detection and noise reduction
– Developing secure encryption methods
– Improving wireless communication protocols
Chaos-based encryption, for instance, leverages the sensitivity of chaotic systems to create highly secure communication channels.
6. Fluid Dynamics and Turbulence
Understanding the behavior of fluids is crucial in many fields, from aerodynamics to oceanography. Nonlinear analysis helps in:
– Modeling turbulent flows
– Optimizing the design of aircraft and ships
– Predicting the behavior of plasmas in fusion reactors
– Studying atmospheric and oceanic circulation patterns
The Navier-Stokes equations, which describe fluid motion, are notorious for their nonlinear complexity and remain one of the great unsolved problems in mathematics.
7. Materials Science and Nanotechnology
At the nanoscale, materials often exhibit nonlinear properties that can be harnessed for various applications:
– Developing new materials with unique properties
– Optimizing manufacturing processes
– Designing more efficient solar cells and batteries
– Creating advanced sensors and actuators
Nonlinear optics, for example, has led to the development of new laser technologies and optical computing devices.
Challenges and Future Directions
While nonlinear analysis has proven immensely valuable, it’s not without its challenges:
1. Computational complexity: Nonlinear systems often require significant computational resources to analyze and simulate.
2. Sensitivity to initial conditions: The chaotic nature of many nonlinear systems makes long-term predictions challenging.
3. Model selection: Choosing the right nonlinear model for a given system can be difficult and often requires domain expertise.
4. Interpretability: Nonlinear models can be more difficult to interpret than their linear counterparts, potentially limiting their applicability in some fields.
Despite these challenges, the future of nonlinear analysis looks bright. Advancements in computing power, machine learning, and data analysis techniques are opening up new possibilities:
- Quantum computing may provide the computational power needed to tackle even more complex nonlinear problems.
- Machine learning algorithms are being developed to automatically identify and model nonlinear relationships in data.
- Interdisciplinary collaborations are leading to novel applications of nonlinear analysis in fields like social sciences and psychology.
Conclusion: Nonlinear analysis has emerged as an indispensable tool for understanding and predicting the behavior of complex systems in our world. From weather forecasting to financial modeling, from biological systems to advanced engineering, nonlinear techniques are helping us unveil the intricate dynamics that shape our reality.
As we continue to push the boundaries of science and technology, the importance of nonlinear analysis will only grow. It offers us a lens through which we can view the world in all its beautiful complexity, reminding us that sometimes, the most profound insights come from embracing the chaos rather than trying to simplify it.
By harnessing the power of nonlinear analysis, we’re not just solving problems – we’re gaining a deeper understanding of the fundamental nature of our universe. And in doing so, we’re opening up new possibilities for innovation, discovery, and progress across all fields of human endeavor.
| Read More Topics |
| Introduction of error control codes |
| Analog link in optical communication |
| Penalty power in optical communication |