Poisson's Ratio (σ) - Pedagogy Zone

When a wire is stretched, the length of the wire will increase. At the same time, the wire will become thinner, that is, there is a decrease in the cross section of the wire. The ratio of change in diameter of the wire to the original diameter of the wire is known as lateral strain. The ratio of change in the length of the wire to the original length is known as longitudinal strain.

Within the elatic limit, the ratio of the lateral strain to the longitudinal strain is known as Poisson’s ratio.

Poisson’s ratio $\quad \sigma \quad=\frac{\text { Lateral strain }}{\text { Longitudinal strain }}$

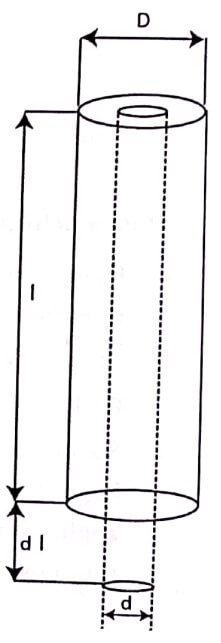
Poissons ratio

\begin{aligned} \text { Lateral strain } & =\frac{\mathrm{d}}{D} \\ \text { Longitudinal strain } & =\frac{\mathrm{d} l}{l} \\ \sigma & =\frac{\mathrm{d} / D}{\mathrm{~d} l / l} \end{aligned}

where $d$ and $dl$ are change in diameter and length respectively and $D$ and $l$ are original diameter and length respectively. If α and β are the longitudinal strain per unit stress and the lateral strain per unit stress respectively, then